Tokyo/Formulation
From 2007.igem.org
(Difference between revisions)
| Line 14: | Line 14: | ||
<br>Introduction | <br>Introduction | ||
<br>First, we saw the qualitative nature implied by simple dimentionless equations and found that the number of the stable points is one or two. Since our model must have A and B states, the number of its stable points should be two. | <br>First, we saw the qualitative nature implied by simple dimentionless equations and found that the number of the stable points is one or two. Since our model must have A and B states, the number of its stable points should be two. | ||
| - | <br>For the parameters required this bistability, we have found that the production rates and Hill coefficients are critical.--> | + | <br>For the parameters required for this bistability, we have found that the production rates and Hill coefficients are critical. |
| + | 上記の英語訳のPhaseplane, Threeは下の日本語訳にないです。 | ||
| + | --> | ||
| + | <!--案2 | ||
| + | <br>Introduction | ||
| + | <br>First, according to the simple dimentionless equations of our toggle, the number of equibrium points is one or two. To takes two states of A and B, our model should have two equibrium points. | ||
| + | --> | ||
== [[Tokyo/Formulation/2.toggle model with hybrid promoter |Step2.toggle model with hybrid promoter ]] == | == [[Tokyo/Formulation/2.toggle model with hybrid promoter |Step2.toggle model with hybrid promoter ]] == | ||
Revision as of 03:52, 22 October 2007
Step1.toggle model
<イントロ> まずは,次元をもっていない単純な式で定性的な振る舞いをみてみる. その結果,安定点が1つのときと2つのときがある.我々が求めているモデルはA状態とB状態をとる必要があるので, 安定点が二つのときである.bistalbeになるには,パラメータにおいて,合成rateの強さ比とヒル係数が重要であることが分かった.
Step2.toggle model with hybrid promoter
<イントロ>
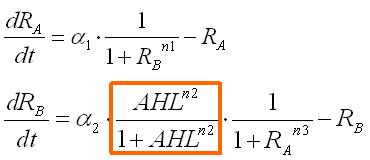
ハイブリットプロモータを式に入れ込む必要がある.
トグルの抑制項に加えてAHLによってactivateされる項が加わる.
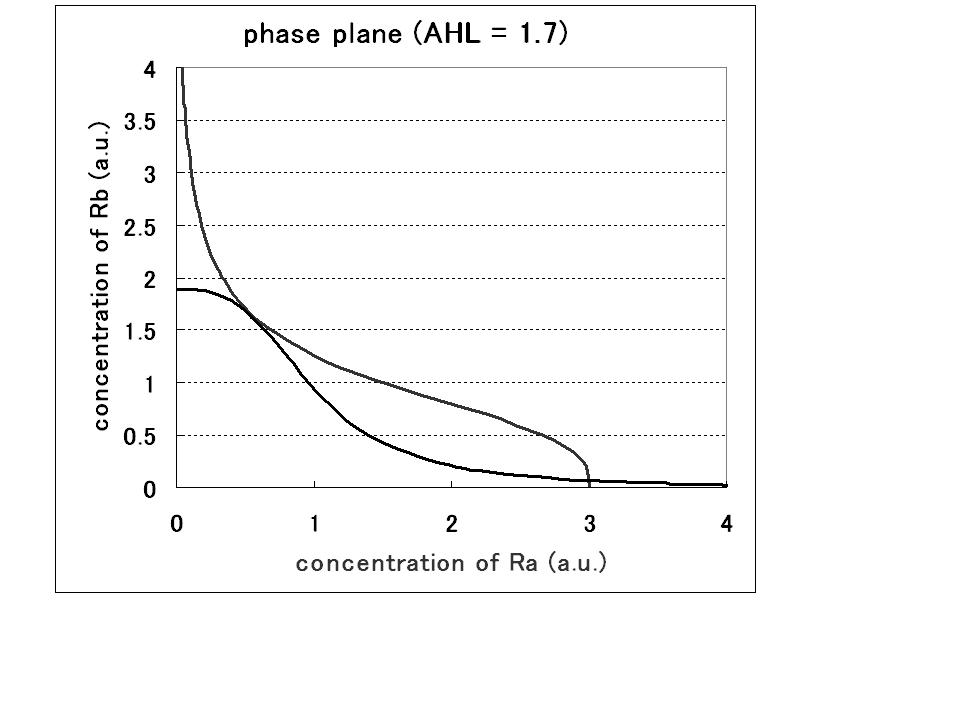
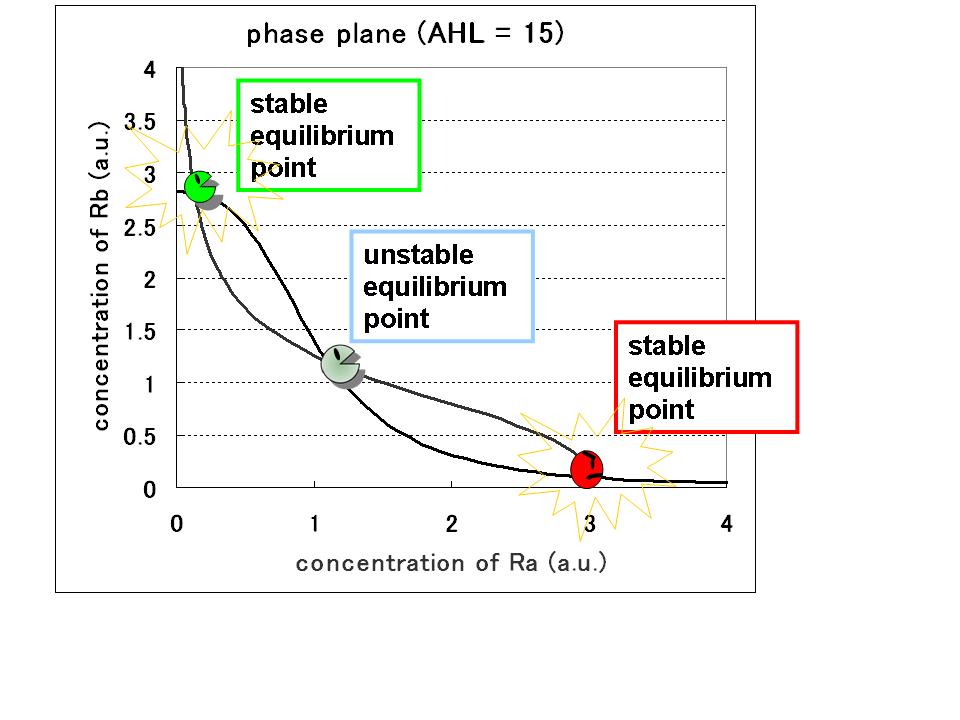
これにより,AHL量によって相平面変化するようになる.
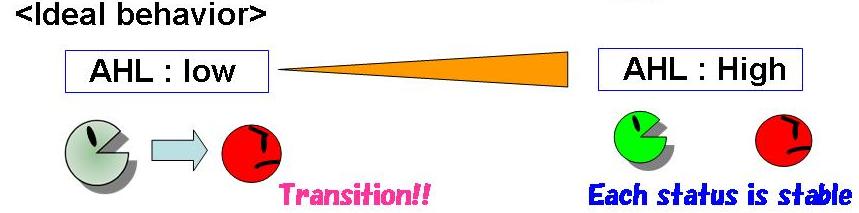
AHL量が少ないときはmonoで多いときトグルと同じになりbiになる.
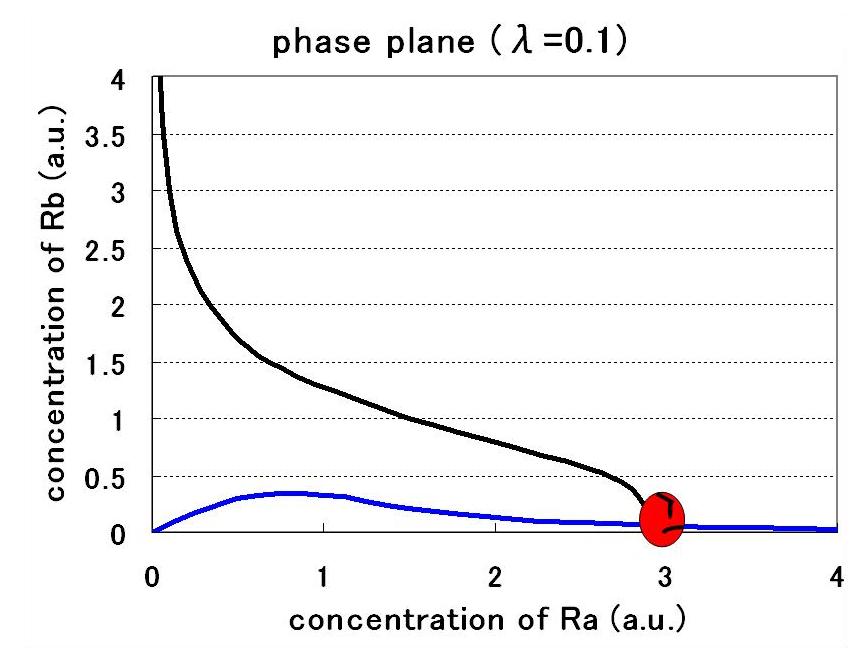
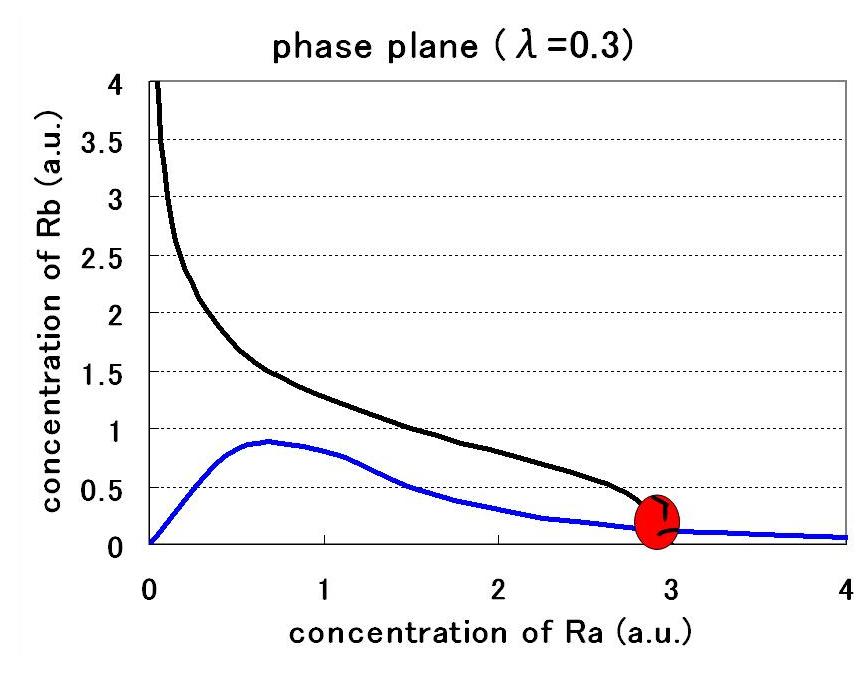
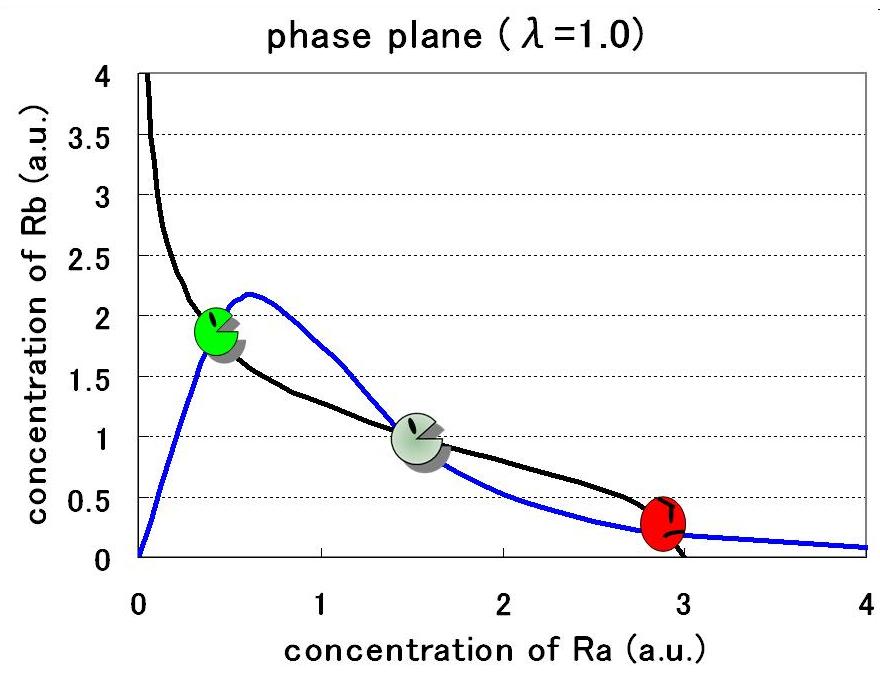
Step3.AHL-experssing model
<イントロ>
今度は,大腸菌の中からAHLを作り出す系に拡張する.
すると,nullclineが非対称になり,従来のトグルとは異なった相平面になる.
この際,新たなパラメータλが入ってくる.
パラメータセットによって,monoになったり,biになったりする.
for more detail
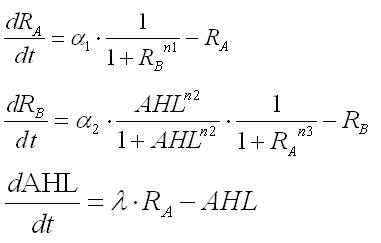
Step4.population model
<イントロ> 1個体から複数個体に拡張.AHLの式は,AHLの移動がfreelyと論文にあるので大腸菌内と外とを区別していない ここでも,相平面解析を行った.n個体の振る舞いであっても,その中の1個体に着目することで相平面解析を可能にした. この際,新たなパラメータとして個体数nがはいってくる.
ただし,deterministicなため全個体同じ動き.我々のモデルはこれではみれない. stochasticなシミュレーションが要求される.