Tristable/Modeling

From 2007.igem.org

(Difference between revisions)
(→Stability)
(→Stability)
Line 146: Line 146:
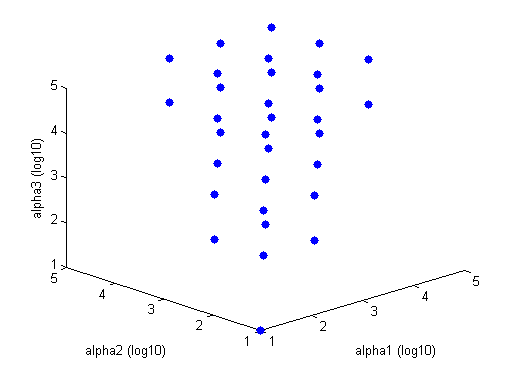
Blue points are stable combinations of repressor production rates, while the rest of the graph is comprised of unstable combinations of repressor production rates.  This graph is for Beta = 2. The tristable region gets larger (i.e. more disparate alpha values will be able to constitute a tristable system) as beta gets larger. The tristable region disappears when Beta equals one or less.[[Image:tri stableRegion.png|left|Tri-Stable region solved in Matlab]]
Blue points are stable combinations of repressor production rates, while the rest of the graph is comprised of unstable combinations of repressor production rates.  This graph is for Beta = 2. The tristable region gets larger (i.e. more disparate alpha values will be able to constitute a tristable system) as beta gets larger. The tristable region disappears when Beta equals one or less.[[Image:tri stableRegion.png|left|Tri-Stable region solved in Matlab]]
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
 +
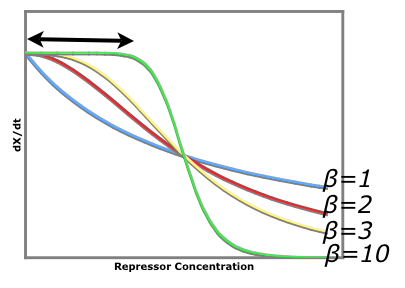
An easy visual aid to seeing how increased beta values (cooperativity of repression) leads to a more stable system consider the following graph of rate of production of repressor1 vs. the repressor that inhibits the production of repressor1. dx/dt vs. [y]
An easy visual aid to seeing how increased beta values (cooperativity of repression) leads to a more stable system consider the following graph of rate of production of repressor1 vs. the repressor that inhibits the production of repressor1. dx/dt vs. [y]

Revision as of 00:15, 25 October 2007

Brown University
Home Lead Sensor Tristable Switch Community Supplemental About us



Contents

Parameters in the Model

Repressor Production Rate

Repressor production rate is determined by two factors:

  1. Promoter Strength (Transcription)
  2. Ribosome Binding Strength (Translation)

In the model, the total repressor production rate = alpha

The promoter strength cannot be easily changed because this would require mutations in the promoter or a different promoter/repressor combo. However, RBSs have been well characterized and the alpha parameter can be modulated by inserting different strength RBSs as determined by the model and testing.

Repressor Strength

The strength of the repressors is determined by:

  1. The repressor concentration, [Repressor].
  2. The cooperativity of repression, Beta.

In the Model, the repressor strength = [Repressor]^Beta

The Beta value is characteristic of each repressor and represents a constant that cannot be changed.

Degradation

The degradation in the model is denoted by the term "-repressor," where the degradation constant is unity because the rates are relative, rather than absolute. The next iteration of the model will include degradation constants. Currently in the registry all of the repressors have strong degradation tags. Jason Kelly made a comment on one repressor and said he was suspicious of how strong of a repression would be able to be obtained from the repessors. We need strong repression for our system, so we may need to remove the tag our system to function.

The Model

Tri-Stable Mathematical Model


  • Beta = Repression Strength
  • Alpha = Repressor Production Rate
  • X = Repressor1
  • Y = Repressor2
  • Z = Repressor3



Stability

Blue points are stable combinations of repressor production rates, while the rest of the graph is comprised of unstable combinations of repressor production rates. This graph is for Beta = 2. The tristable region gets larger (i.e. more disparate alpha values will be able to constitute a tristable system) as beta gets larger. The tristable region disappears when Beta equals one or less.
Tri-Stable region solved in Matlab











An easy visual aid to seeing how increased beta values (cooperativity of repression) leads to a more stable system consider the following graph of rate of production of repressor1 vs. the repressor that inhibits the production of repressor1. dx/dt vs. [y]

Comparison of different beta values

The black arrow shows that there is a larger region for larger beta values where stray repessors will not significantly affect the rate of repressor production rate whereas in a system of beta = 1, stray repressors will significantly change the system, making it less robust.

Modeling

The first draft of the code started last year:

[http://parts2.mit.edu/wiki/index.php/Table_of_preliminary_model_constants Initial Table of Constants]

[http://parts2.mit.edu/wiki/index.php/Derivation_of_the_Model_Equations Derivation of Model Equations]

Media:tristable2006.txt This code proved to complicated to work with and a more simplified version was developed.


A simpler model based on the bistable paper was developed that takes the combined, relative transcription/translation rates into account Media:tristable2007.txt.

Retrieved from "http://2007.igem.org/wiki/index.php/Tristable/Modeling"